Energy storage properties of NaNbO3-based lead-free superparaelectrics with large antiferrodistortion
Abstract
NaNbO3-based lead-free energy storage ceramics are essential candidates for next-generation pulsed power capacitors, especially under the background of energy saving and environmental protection. However, the room-temperature antiferroelectric P phase of pure NaNbO3 ceramics limits its further development in energy storage owing to the irreversible antiferroelectric to ferroelectric phase transition under electric fields. In this work, CaZrO3 was introduced to NaNbO3 ceramics to destroy the long-range polarization ordering but keep large antiferrodistortion, causing the formation of superparaelectric state with macrodomains, which can be identified by the refinement results of high-energy synchrotron X-ray diffraction, neutron diffraction and TEM results. Combined with the fine grains, dense and homogeneous microstructure, ergodic relaxation behaviors, and delayed polarization saturation, a high recoverable energy storage density of ~5.4 J/cm3 and efficiency of ~82% can be realized in 0.85NaNbO3-0.15CaZrO3 ceramics at an ultrahigh breakdown electric field of ~68 kV/mm. The results found in this work suggest that the supersparaelectric with non-cubic phase would be a good candidate for generating excellent dielectric energy storage properties.
Keywords
INTRODUCTION
Energy plays an irreplaceable role in the development of human society, and how to efficiently store energy has gradually become the focus of research. Currently, energy storage devices are mainly divided into four categories: lithium-ion batteries, fuel cells, electrochemical super-capacitors, and dielectric capacitors[1-3]. Solid-state dielectric capacitors, compared with other energy storage devices, possess high power density and ultrafast charge-discharge rates, which are widely used in advanced high power and pulse power electronic devices, such as hybrid electric vehicles, distributed power systems, and directional energy weapons[4,5]. However, the low recoverable energy storage density (Wrec) limits their energy storage development.
In the context of energy saving and environmental protection, to effectively improve the Wrec of dielectric capacitors, lead-free perovskite energy storage ceramics have become a research hotspot[6,7]. The total energy storage density (Wtotal), Wrec, and efficiency (η) are the main parameters to evaluate energy storage performance which can be calculated based on the following formula:
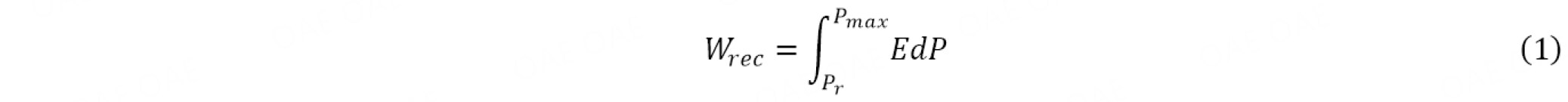
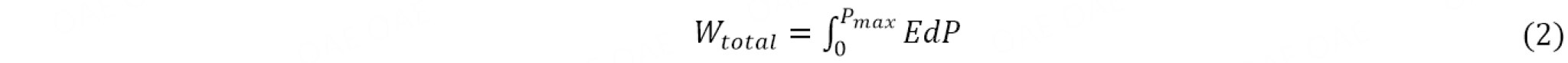
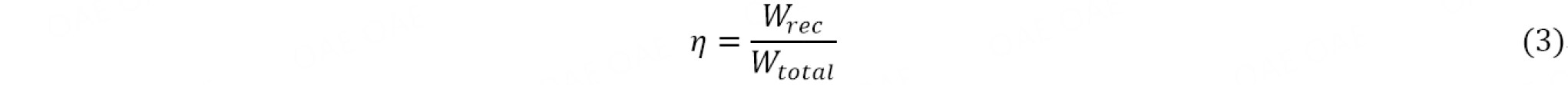
where Pmax, Pr, and E are the maximum polarization, remanent polarization, and applied electric field, respectively. As a result, large ∆P (Pmax-Pr) and high Eb are indispensable for materials with high Wrec[8]. The researches on lead-free energy storage materials generally focus on linear dielectrics, ferroelectrics, antiferroelectrics, and relaxor ferroelectrics. Linear dielectrics possess ultrahigh η and Eb but low Wrec due to their low polarization characteristic[9]. Both high Pmax and Pr can be found in ferroelectrics, resulting in highly inferior Wrec and η. Similarly, antiferroelectrics also own unsatisfactory energy storage properties with low η and poor cycle stability because of irreversible antiferroelectric to ferroelectric phase transition under applied electric field and comparatively significant difference between EF and EA[10-12]. Relaxor ferroelectrics are characterized by a diffuse phase transition over a broad temperature range, from the Burns temperature (TB) at which nanodomains appear, to the intermediate temperature (Tm) at which nanodomains grow and the permittivity reaches the maximum, and finally to the freezing temperature (Tf) at which nanodomains become frozen(Tf < Tm < TB)[13,14]. In particular, relaxor ferroelectrics located at the temperature range of
NaNbO3 (NN) is one of the typical lead-free ferroelectrics with complex crystal structure and phase transition under various temperatures. Despite the remaining controversies, it is commonly agreed that NN adopts seven major phases with the sequence of U→T2→T1→S→R→P→N on cooling, where the common P and R phases are antiferroelectrics[21,22]. The complex temperature-driven structure also means great potential for performance regulation. NN ceramic exhibits antiferroelectric P phase structure with Pbma space group at room temperature[23,24]. Generally, an effective strategy to improve the energy storage of NN ceramics focuses on stabilizing their antiferroelectric phase. For example, ultrahigh Wrec of 12.2 J/cm3 was obtained in 0.76NaNbO3-0.24(Bi0.5N0.5)TiO3 ceramics due to stable relaxor antiferroelectric phase, however, accompanied by a relatively low η of 69% owing to large polarization hysteresis for the first-order antiferroelectric-ferroelectric phase transition under high electric field[25]. Therefore, a practical approach is urgently required to simultaneously regulate the Wrec and η of NN ceramics. In this work, CaZrO3 (CZ) was introduced into NN ceramics to not only destroy long-range antiferroelectric ordering but also remain large antiferrodistortion. On the one hand, the enhanced local random field along with the strengthened dielectric relaxation behavior would benefit the high η owing to the fast response of nanoclusters to the external electric field. On the other hand, the existence of large oxygen octahedron tilt would hinder the formation of long-range ferroelectric ordering under electric field, leading to the delayed polarization saturation process. Combined with the fine grains, dense and homogeneous microstructure, ergodic relaxation behavior, and delayed polarization saturation, a high recoverable energy storage density of
MATERIALS AND METHODS
Sample preparation
The ceramics of (1-x)NaNbO3-xCaZrO3 ((1-x)NN-xCZ, x = 0-0.15) were prepared by the conventional solid-state reaction process. The raw materials of Na2CO3 (> 99.9%), CaCO3 (> 99.5%), Nb2O5 (> 99.9%), and ZrO2 (> 99.9%) were weighed according to the chemical formula and mixed by planetary ball milling for 8 h using ethanol as ball milling media. The mixed powders were calcined at 850 °C for 5 h after drying. Then, the calcined powders were ball-milled again by high-energy ball milling (700 r/min for 8 h) with ethanol and 0.5 wt% PVB binder. Afterward, the powders were pressed into pellets with a diameter of 8 mm and a thickness of ~1 mm. The pellets were sheathed using the corresponding calcined powders in crucibles and sintered at 1370 °C for 2 h. Finally, the sintered ceramics were polished to a thickness of ~0.1 mm with a diameter of ~6.5 mm and coated with silver electrodes with a diameter of ~2 mm, which were fired under 550 °C for 30 min to measure their electrical properties.
Structural and performance characterizations
The high-energy synchrotron X-ray diffraction (SXRD) data was measured on the 11-ID-C beamline of advanced photon source. Powder neutron diffraction data were collected at CSNS (China Spallation Neutron Source, MPI) using time-of-flight powder diffractometers. The diffraction data refinement was taken by the Rietveld method on software GSAS II. Temperature- and frequency-dependent dielectric properties were carried out using an impedance analyzer (Keysight E4990A, Santa Clara, CA). Domain morphology and selected area electron diffraction (SAED) were observed on a field-emission transmission electron microscope (TEM, JEM-F200, JEOL, Japan) at an accelerating voltage of 200 kV. High-angle annular dark-field (HAADF) atomic-scale images were obtained using an atomic-resolution scanning transmission microscope (STEM, aberration-corrected Titan Themis 3300), and the polarization vectors, polarization magnitude, and polarization angle maps were calculated by customized MATLAB scripts. The morphology of grains was filmed using a scanning electron microscope (SEM, LEO1530, ZEISS SUPRA 55, Oberkochen, Germany). Energy-storge properties of ceramics were investigated by a ferroelectric analyzer (aix ACCT, TF Analyzer 1000, Aachen, Germany).
RESULTS AND DISCUSSION
Figure 1A shows the temperature-dependent dielectric permittivity (εr) of (1-x)NN-xCZ ceramics at 1 MHz. Pure NN is determined to be antiferroelectric P phase structure at room temperature accompanied by two dielectric anomaly peaks at 130 °C and 370 °C, representing the transitions from antiferroelectric P phase to incommensurate (INC) phase and INC phase to antiferroelectric R phase, respectively[26-28]. With the increase of CZ, the dielectric anomaly peak at 130 °C disappears, and the maximum dielectric peak shifts gradually to low temperature together with the transition of phase structure. To characterize the relaxor feature, the dielectric properties of x = 0.1 and 0.12 at different frequencies are shown in Figure 1B. Both samples exhibit apparent frequency dispersion behavior. As shown in Figure 1C, the diffuseness degree (γ) for x = 0.1 and 0.12 was obtained using the modified Curie-Weiss Law:
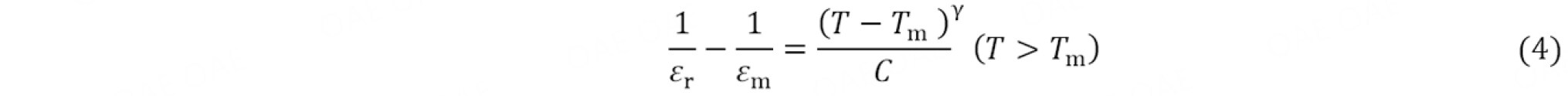
Figure 1. (A) Temperature-dependent dielectric permittivity for (1-x)NN-xCZ ceramics. (B) Frequency-dependent dielectric permittivity and (C) diffuseness degree (γ) fitted from the modified Curie-Weiss law for x = 0.1 and 0.12. (D) SEM surface morphology and grain size distribution of 0.85NN-0.15CZ ceramic.
where εm is the maximum dielectric permittivity and Tm is the according temperature, C is the Curie constant. The γ value of 0.9NN-0.1CZ and 0.88NN-0.12CZ ceramics are ~1.41 and ~1.92, respectively, indicating that the (1-x)NN-xCZ ceramics should be relaxor ferroelectrics for x ≥ 0.1. These also demonstrate that the Ca2+ and Zr4+ are substituted into the lattice of NN matrix, breaking the long-range antiferroelectric order and increasing the local random field. Especially, the relaxed dielectric peak of 0.85NN-0.15CZ ceramic located far below room temperature and the TB ~85 °C obtained according to the Curie-Weiss Law, as shown in Supplementary Figure 1, indicate it should be superparaelectric state around room temperature. It is recognized that ultrasmall and highly active polar nanoregions (PNRs) can be found in the superparaelectric region, leading to the improvement of η[29-31]. Compared with other samples, 0.85NN-0.15CZ ceramic has moderate room-temperature εr ~545, which can effectively delay the polarization saturation process under a low electric field and reduce the possibility of electromechanical breakdown generated by the electrostriction effect. These phenomena indicate that 0.85NN-0.15CZ ceramic shows excellent potential to become a high energy storage material.
Another basic guarantee for realizing high energy storage properties is the uniform and compact microstructure. As shown in Figure 1D, the surface morphology of 0.85NN-0.15CZ ceramic presents a dense microstructure with few pores. A uniform grain size distribution can be found in 0.85NN-0.15CZ ceramic with a smaller average grain size (Ga) of ~2.1 μm compared with that of pure NN ceramic shown in Supplementary Figure 2. Moreover, the uniform distribution of elements in Supplementary Figure 3 suggests the achievement of a pure phase structure. It is believed that good sample quality, along with small grain size and dense structure, is beneficial to strengthening Eb.
Even though a superparaelectric state for the 0.85NN-0.15CZ ceramic can be easily identified by using dielectric spectra, however, it is widely known that there are several different paraelectric states as well as (anti)ferroelectric states in NN ceramics at different temperature ranges. To analyze the phase structure of 0.85NN-0.15CZ ceramic, as shown in Figure 2A and B, high-energy SXRD and powder neutron diffraction data were collected and refined. Together with the EDS images shown in Supplementary Figure 3, the sample should certainly be a pure perovskite phase. Moreover, apparent non-cubic phase structure can be identified for 0.85NN-0.15CZ ceramic according to the split main diffraction peaks and superlattice diffraction peaks. This feature is quite different from the average structure characteristics of traditional superparaelectrics[15,16], indicating the existence of lattice distortion. The lattice distortion in (anti)ferroelectrics mainly includes oxygen octahedron tilt and cation off-centering displacement. Considering the macro nonpolar feature of superparaelectrics, the lattice distortion in the studied sample should be attributed to the oxygen octahedron tilt. As the insensitive response of X-ray to the oxygen ions, powder neutron diffraction was measured. Rietveld refinement using the model with P21ma space group was taken simultaneously on the SXRD and neutron diffractions, and the satisfying results with low-reliability factors of weighted patterns (Rwp) are shown in Figure 2A and B. To convince the best refinement result, Rietveld refinement of SXRD data using the model with cubic space group of Pm-3m was also carried out in Supplementary Figure 4. It can be found that the 0.85NN-0.15CZ ceramic should be a ferroelectric Q phase with P21ma space group and a-b+c- oxygen octahedron tilt system but small polarization displacement, which is different from that of NN ceramic (P phase: a-b-c-/a-b+c-). According to Glazer notation, the superscripts +, -, and 0 represent in-phase tilt, anti-phase tilt, and no tilt of oxygen octahedral along one axis, respectively[32]. The tilt degree of oxygen octahedron can be calculated using ω = (180°-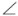 B-O-B)/2. As shown in Supplementary Figure 5, the oxygen octahedron tilt degree for NN ceramics with Pbcm space group at room temperature is calculated as ~7.9°-13.15°. According to the lattice parameters obtained from the refinement results of SXRD and powder neutron diffraction, the crystal structure model of 0.85NN-0.15CZ ceramic was drawn and displayed in Figure 2C. A large oxygen octahedron tilt of ~10.89°-12.20° can be calculated according to the
B-O-B)/2. As shown in Supplementary Figure 5, the oxygen octahedron tilt degree for NN ceramics with Pbcm space group at room temperature is calculated as ~7.9°-13.15°. According to the lattice parameters obtained from the refinement results of SXRD and powder neutron diffraction, the crystal structure model of 0.85NN-0.15CZ ceramic was drawn and displayed in Figure 2C. A large oxygen octahedron tilt of ~10.89°-12.20° can be calculated according to the 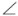 B-O1-B ~157.55°,
B-O1-B ~157.55°, 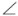 B-O2-B ~156.61°,
B-O2-B ~156.61°, 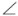 B-O3-B ~155.61°, and
B-O3-B ~155.61°, and  B-O4-B ~158.22°, which is much larger than that of traditional relaxor ferroelectrics such as Pb(Mg1/3Nb2/3)O3 and Ba(Ti, Zr)O3. That is to say, the relaxor ferroelectric Q phase can be identified in 0.85NN-0.15CZ ceramic, which is quite different from the previously reported results that the addition of CZ would stabilize antiferroelectric P phase in NN ceramic[33-35]. The decreased tolerance factor after doping CZ into NN ceramic would increase the oxygen octahedron tilt. However, according to the statistics of recently reported antiferroelectrics (NN, AgNbO3, (Bi0.5Na0.5)TiO3-based, BiFeO3-based, PbZrO3, and PbHfO3-based ceramics), it can be found that the antiferroelectric phase only exists in a narrow tolerance factor range. The perovskites with ultralow tolerance factor are usually paraelectrics, such as CaZrO3 and CaHfO3. Therefore, the polarization ordering would be destroyed when the amount of CZ is over a critical value, leading to the disappearance of anti-phase tilt modules along b axis. Moreover, the formation of dielectric relaxation behavior would also destroy the long-range polarization ordering. As a result, a superparaelectric state with large antiferrodistortion can be detected in 0.85NN-0.15CZ ceramic at room temperature.
B-O4-B ~158.22°, which is much larger than that of traditional relaxor ferroelectrics such as Pb(Mg1/3Nb2/3)O3 and Ba(Ti, Zr)O3. That is to say, the relaxor ferroelectric Q phase can be identified in 0.85NN-0.15CZ ceramic, which is quite different from the previously reported results that the addition of CZ would stabilize antiferroelectric P phase in NN ceramic[33-35]. The decreased tolerance factor after doping CZ into NN ceramic would increase the oxygen octahedron tilt. However, according to the statistics of recently reported antiferroelectrics (NN, AgNbO3, (Bi0.5Na0.5)TiO3-based, BiFeO3-based, PbZrO3, and PbHfO3-based ceramics), it can be found that the antiferroelectric phase only exists in a narrow tolerance factor range. The perovskites with ultralow tolerance factor are usually paraelectrics, such as CaZrO3 and CaHfO3. Therefore, the polarization ordering would be destroyed when the amount of CZ is over a critical value, leading to the disappearance of anti-phase tilt modules along b axis. Moreover, the formation of dielectric relaxation behavior would also destroy the long-range polarization ordering. As a result, a superparaelectric state with large antiferrodistortion can be detected in 0.85NN-0.15CZ ceramic at room temperature.
Figure 2. (A) High-energy synchrotron XRD, and (B) powder neutron diffraction structure refinement patterns of 0.85NN-0.15CZ Ceramic. (C) Schematic diagram of the large antiferrodistortion in 0.85NN-0.15CZ ceramic.
TEM is an essential and helpful method to characterize the domain morphology and lattice microstructure for ferroelectric materials. Figure 3A suggests that 0.85NN-0.15CZ ceramic exhibits apparent 90° and 180° microdomains. Figure 3B and C display high-resolution TEM lattice fringe images along [100]c and [111]c directions, respectively, demonstrating good sample quality. Figure 3D and E show the SAED image along [100]c and [111]c directions, respectively, which once again confirm that 0.85NN-0.15CZ ceramic should be pure perovskite structure. It is recognized that the 1/2 types of superlattice diffractions of (ooe)/2 and (ooo)/2 (o and e are odd and even, respectively) are mainly related to the in-phase and anti-phase oxygen octahedron tilt, respectively[32]. Therefore, the 1/2 type superlattice diffraction spots observed in the accordingly SAED images further prove the existence of oxygen octahedral distortion. It is widely accepted that normal ferroelectrics with macrodomains exhibit poor energy storage properties ascribed to the large polarization hysteresis along with irreversible domain switching, which occurs together with polarization reorientation. However, there is no macroscopic polarization alignment in this superparaelectric sample, which could be further directly confirmed by the quantitative analysis of the polarization mapping using HAADF-STEM results, as shown in Figure 4. Therefore, these macrodomains should be ferroelastic domains constructed by the ordered oxygen octahedron tilt, and the domain switching process has very little influence on polarization reorientation. According to the polarization mapping, ultrasmall PNRs with a size of about 2-3 nm can be seen, which is at the same level as other superparaelectrics. Namely, fast response of PNRs with little polarization hysteresis during charging and discharging processes can also be expected for this sample, benefiting excellent energy storage properties. Moreover, large antiferrodistortion would also delay the polarization saturation process, which would also favor the energy storage properties.
Figure 3. (A) Domain morphology of 0.85NN-0.15CZ ceramic. Lattice fringes and SAED patterns of 0.85NN-0.15CZ ceramic along
Figure 4. (A) Atomic-resolution HAADF-STEM polarization vector image and (B) polarization displacement distribution mappings along [100]c.
Due to the irreversible phase transition from antiferroelectric to ferroelectric under electric fields for pure NN ceramic, a square P-E loop with poor energy storage properties can be achieved, as shown in Supplementary Figure 6. With the stabilization of antiferroelectric P phase by adding a small content of CZ[35], even though increased energy storage density can be obtained along with the appearance of repeatable double P-E loop, quite low energy efficiency can also be found owing to the large hysteresis caused by the first order antiferroelectric-ferroelectric phase transition. An obvious increase in both energy storage density and efficiency can be detected with the entrance of relaxor ferroelectric phase zone of 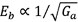 [36], small grain size is favorable for the enhancement of Eb. Complex impedance spectroscopy of pure NN and 0.85NN-015CZ ceramics measured at 500 °C are shown in
[36], small grain size is favorable for the enhancement of Eb. Complex impedance spectroscopy of pure NN and 0.85NN-015CZ ceramics measured at 500 °C are shown in
Figure 5. (A) P-E loops and energy storage performance under various electric fields for 0.85NN-0.15CZ ceramics. (B) Comparison of energy storage performance among 0.85NN-0.15CZ ceramic and some other systems[6].
Advanced ceramic capacitors are developing toward large energy storage density and high efficiency[37]. Figure 5B shows the comparison of energy-storage performance among 0.85NN-0.15CZ ceramic and other relevant dielectric energy storage ceramics (AgNbO3(AN), BiFeO3(BF), Bi0.5K0.5TiO3(BKT), Bi0.5Na0.5TiO3(BNT), BaTiO3(BT), K0.5Na0.5NbO3(KNN), SrTiO3(ST))[6,12,18,38-46]. Obviously, 0.85NN-0.15CZ ceramic exhibits great performance superiority, making it one of the prospective materials for advanced pulse power capacitor applications.
CONCLUSIONS
In this work, (1-x)NN-xCZ ceramics are prepared by a conventional solid-state reaction method. With increasing CZ content to 0.15, the structure of samples changes from antiferroelectric P phase to relaxor ferroelectric Q phase with superparaelectric state, leading to the destruction of long-range polarization ordering but reservation of antiferrodistortion ordering, which can be confirmed by the high energy synchrotron XRD and powder neutron diffraction refinement results as well as TEM images. In this case, the grain size of the sample decreases to 2.1 μm, accompanied by dense and homogeneous microstructure. The 0.85NN-0.15CZ ceramics showed comprehensive energy storage performance of Wrec = 5.4 J/cm3 and
DECLARATIONS
Authors’ contributions
Experiment, characterization, writing original draft: Liu G
Review & editing, supervision: Qi H, Chen L
Conceptualization, review, supervision: Qi H
Availability of data and materials
Not applicable.
Financial support and sponsorship
This work was supported by the National Natural Science Foundation of China (Grant Nos. 52172181 and 22105017).
Conflicts of interest
All authors declared that there are no conflicts of interest.
Ethical approval and consent to participate
Not applicable.
Consent for publication
Not applicable.
Copyright
© The Author(s) 2023.
Supplementary Materials
REFERENCES
1. Hao X, Zhai J, Kong LB, Xu Z. A comprehensive review on the progress of lead zirconate-based antiferroelectric materials. Prog Mater Sci 2014;63:1-57.
2. Qi H, Xie A, Zuo R. Local structure engineered lead-free ferroic dielectrics for superior energy-storage capacitors: a review. Energy Stor Mater 2022;45:541-67.
3. Yang L, Kong X, Li F, et al. Perovskite lead-free dielectrics for energy storage applications. Prog Mater Sci 2019;102:72-108.
4. Wang G, Lu Z, Li Y, et al. Electroceramics for high-energy density capacitors: current status and future perspectives. Chem Rev 2021;121:6124-72.
5. Li J, Shen Z, Chen X, et al. Grain-orientation-engineered multilayer ceramic capacitors for energy storage applications. Nat Mater 2020;19:999-1005.
6. Yang Z, Du H, Jin L, Poelman D. High-performance lead-free bulk ceramics for electrical energy storage applications: design strategies and challenges. J Mater Chem A 2021;9:18026-85.
7. Yao FZ, Yuan Q, Wang Q, Wang H. Multiscale structural engineering of dielectric ceramics for energy storage applications: from bulk to thin films. Nanoscale 2020;12:17165-84.
8. Veerapandiyan V, Benes F, Gindel T, Deluca M. Strategies to improve the energy storage properties of perovskite lead-free relaxor ferroelectrics: a review. Materials 2020;13:5742.
9. Guo X, Pu Y, Wang W, et al. Ultrahigh energy storage performance and fast charge-discharge capability in Dy- modified SrTiO3 linear ceramics with high optical transmissivity by defect and interface engineering. Ceram Int 2020;46:21719-27.
10. Xie A, Qi H, Zuo R, Tian A, Chen J, Zhang S. An environmentally-benign NaNbO3 based perovskite antiferroelectric alternative to traditional lead-based counterparts. J Mater Chem C 2019;7:15153-61.
11. Dong X, Li X, Chen X, et al. High energy storage density and power density achieved simultaneously in NaNbO3-based lead-free ceramics via antiferroelectricity enhancement. J Materiomics 2021;7:629-39.
12. Tian A, Zuo R, Qi H, Shi M. Large energy-storage density in transition-metal oxide modified NaNbO3-Bi(Mg0.5Ti0.5)O3 lead-free ceramics through regulating the antiferroelectric phase structure. J Mater Chem A 2020;8:8352-9.
15. Pan H, Lan S, Xu S, et al. Ultrahigh energy storage in superparaelectric relaxor ferroelectrics. Science 2021;374:100-104.
16. Chen L, Wang N, Zhang Z, et al. Local diverse polarization optimized comprehensive energy-storage performance in lead-free superparaelectrics. Adv Mater 2022;34:e2205787.
17. Yang W, Zeng H, Yan F, et al. Superior energy storage properties in NaNbO3-based ceramics via synergistically optimizing domain and band structures. J Mater Chem A 2022;10:11613-24.
18. Qi H, Zuo R. Linear-like lead-free relaxor antiferroelectric (Bi0.5Na0.5)TiO3-NaNbO3 with giant energy-storage density/efficiency and super stability against temperature and frequency. J Mater Chem A 2019;7:3971-8.
19. Ye H, Yang F, Pan Z, et al. Significantly improvement of comprehensive energy storage performances with lead-free relaxor ferroelectric ceramics for high-temperature capacitors applications. Acta Mater 2021;203:116484.
20. Kang R, Wang Z, Lou X, et al. Energy storage performance of Bi0.5Na0.5TiO3-based relaxor ferroelectric ceramics with superior temperature stability under low electric fields. Chem Eng J 2021;410:128376.
21. Glazer AM, Megaw HD. Studies of the lattice parameters and domains in the phase transitions of NaNbO3. Acta Crystallogr A 1973;29:489-95.
23. Megaw HD, Wells M. The space group of NaNbO3 and (Na0.995K0.005)NbO3. Acta Crystallogr 1958;11:858-62.
24. Guo H, Shimizu H, Mizuno Y, Randall CA. Strategy for stabilization of the antiferroelectric phase (Pbma) over the metastable ferroelectric phase (P21ma) to establish double loop hysteresis in lead-free (1-x)NaNbO3-xSrZrO3 solid solution. J Appl Phys 2015;117:214103.
25. Qi H, Zuo R, Xie A, et al. Ultrahigh energy-storage density in NaNbO3-based lead-free relaxor antiferroelectric ceramics with nanoscale domains. Adv Funct Mater 2019;29:1903877.
26. Guo H, Shimizu H, Randall CA. Direct evidence of an incommensurate phase in NaNbO3 and its implication in NaNbO3-based lead-free antiferroelectrics. Appl Phys Lett 2015;107:112904.
27. Reznichenko LA, Shilkina LA, Gagarina ES, et al. Structural instabilities, incommensurate modulations and P and Q phases in sodium niobate in the temperature range 300-500 K. Crystallogr Rep 2003;48:448-56.
28. Wang X, Shen Z, Hu Z, Qin L, Tang S, Kuok M. High temperature Raman study of phase transitions in antiferroelectric NaNbO3. J Mol Struct 1996;385:1-6.
29. Bokov AA, Ye Z. Recent progress in relaxor ferroelectrics with perovskite structure. J Mater Sci 2006;41:31-52.
30. Hu Q, Tian Y, Zhu Q, et al. Achieve ultrahigh energy storage performance in BaTiO3-Bi(Mg1/2Ti1/2)O3 relaxor ferroelectric ceramics via nano-scale polarization mismatch and reconstruction. Nano Energy 2020;67:104264.
31. Yan F, Zhou X, He X, et al. Superior energy storage properties and excellent stability achieved in environment-friendly ferroelectrics via composition design strategy. Nano Energy 2020;75:105012.
33. Guo H, Shimizu H, Randall CA. Microstructural evolution in NaNbO3-based antiferroelectrics. J Appl Phys 2015;118:174107.
34. Liu Z, Lu J, Mao Y, Ren P, Fan H. Energy storage properties of NaNbO3-CaZrO3 ceramics with coexistence of ferroelectric and antiferroelectric phases. J Eur Ceram Soc 2018;38:4939-45.
35. Shimizu H, Guo H, Reyes-Lillo SE, Mizuno Y, Rabe KM, Randall CA. Lead-free antiferroelectric: xCaZrO3-(1-x)NaNbO3 system (0 ≤ x ≤ 0.10). Dalton Trans 2015;44:10763-72.
36. Tunkasiri T, Rujijanagul G. Dielectric strength of fine grained barium titanate ceramics. J Mater Sci Lett 1996;15:1767-9.
37. Luo N, Han K, Cabral MJ, et al. Constructing phase boundary in AgNbO3 antiferroelectrics: pathway simultaneously achieving high energy density and efficiency. Nat Commun 2020;11:4824.
38. Chao W, Gao J, Yang T, Li Y. Excellent energy storage performance in La and Ta co-doped AgNbO3 antiferroelectric ceramics. J Eur Ceram Soc 2021;41:7670-7.
39. Chen J, Qi H, Zuo R. Realizing Stable Relaxor Antiferroelectric and Superior Energy Storage Properties in (Na1-x/2Lax/2)(Nb1-xTix)O3 Lead-Free Ceramics through A/B-Site Complex Substitution. ACS Appl Mater Interfaces 2020;12:32871-9.
40. Lu Z, Bao W, Wang G, et al. Mechanism of enhanced energy storage density in AgNbO3-based lead-free antiferroelectrics. Nano Energy 2021;79:105423.
41. Li S, Hu T, Nie H, et al. Giant energy density and high efficiency achieved in silver niobate-based lead-free antiferroelectric ceramic capacitors via domain engineering. Energy Stor Mater 2021;34:417-26.
42. Guo B, Yan Y, Tang M, et al. Energy storage performance of Na0.5Bi0.5TiO3 based lead-free ferroelectric ceramics prepared via non-uniform phase structure modification and rolling process. Chem Eng J 2021;420:130475.
43. Chen H, Shi J, Dong X, et al. Enhanced thermal and frequency stability and decent fatigue endurance in lead-free NaNbO3-based ceramics with high energy storage density and efficiency. J Materiomics 2022;8:489-97.
44. Pang F, Chen X, Sun C, et al. Ultrahigh energy storage characteristics of sodium niobate-based ceramics by introducing a local random field. ACS Sustain Chem Eng 2020;8:14985-95.
45. Zhu C, Cai Z, Luo B, et al. Multiphase engineered BNT-based ceramics with simultaneous high polarization and superior breakdown strength for energy storage applications. ACS Appl Mater Interfaces 2021;13:28484-92.
Cite This Article
How to Cite
Liu, G.; Chen, L.; Qi, H. Energy storage properties of NaNbO3-based lead-free superparaelectrics with large antiferrodistortion. Microstructures. 2023, 3, 2023009. http://dx.doi.org/10.20517/microstructures.2022.29
Download Citation
Export Citation File:
Type of Import
Tips on Downloading Citation
Citation Manager File Format
Type of Import
Direct Import: When the Direct Import option is selected (the default state), a dialogue box will give you the option to Save or Open the downloaded citation data. Choosing Open will either launch your citation manager or give you a choice of applications with which to use the metadata. The Save option saves the file locally for later use.
Indirect Import: When the Indirect Import option is selected, the metadata is displayed and may be copied and pasted as needed.
































Comments
Comments must be written in English. Spam, offensive content, impersonation, and private information will not be permitted. If any comment is reported and identified as inappropriate content by OAE staff, the comment will be removed without notice. If you have any queries or need any help, please contact us at support@oaepublish.com.